
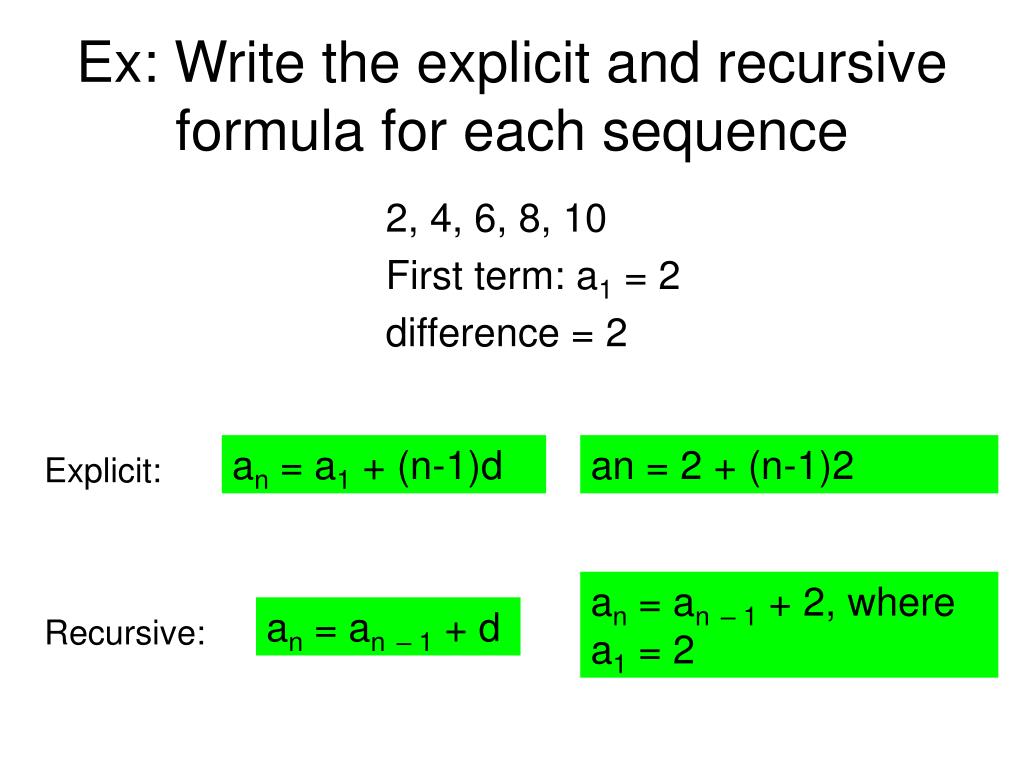
- #Explicit formula for arithmetic sequence guided notes how to
- #Explicit formula for arithmetic sequence guided notes code
Thus 3 ÷ 4 = 3 × 1 / 4 in other words, the quotient of 3 and 4 equals the product of 3 and 1 / 4. For example, in computer algebra, this allows one to handle fewer binary operations, and makes it easier to use commutativity and associativity when simplifying large expressions (for more, see Computer algebra § Simplification). In some contexts, it is helpful to replace a division with multiplication by the reciprocal (multiplicative inverse) and a subtraction by addition of the opposite (additive inverse). The commutative and associative laws of addition and multiplication allow adding terms in any order, and multiplying factors in any order-but mixed operations obey the standard order of operations. Whether inside parenthesis or not, the operator that is higher in the above list should be applied first.

This means that to evaluate an expression, one first evaluates any sub-expression inside parentheses, working inside to outside if there is more than one set. The order of operations, that is, the order in which the operations in an expression are usually performed, results from a convention adopted throughout mathematics, science, technology and many computer programming languages. Most of these ambiguous expressions involve mixed division and multiplication, where there is no general agreement about the order of operations. Internet memes sometimes present ambiguous infix expressions that cause disputes and increase web traffic. When functional or Polish notation are used for all operations, the order of operations results from the notation itself. These rules are meaningful only when the usual notation (called infix notation) is used. If multiple pairs of parentheses are required in a mathematical expression (such as in the case of nested parentheses), the parentheses may be replaced by brackets or braces to avoid confusion, as in − 5 = 9. For example, (2 + 3) × 4 = 20 forces addition to precede multiplication, while (3 + 5) 2 = 64 forces addition to precede exponentiation. Where it is desired to override the precedence conventions, or even simply to emphasize them, parentheses ( ) can be used. These conventions exist to avoid notational ambiguity while allowing notation to remain brief.

When exponents were introduced in the 16th and 17th centuries, they were given precedence over both addition and multiplication and placed as a superscript to the right of their base. Thus, in the expression 1 + 2 × 3, the multiplication is performed before addition, and the expression has the value 1 + (2 × 3) = 7, and not (1 + 2) × 3 = 9. Calculators generally perform operations with the same precedence from left to right, but some programming languages and calculators adopt different conventions.įor example, multiplication is granted a higher precedence than addition, and it has been this way since the introduction of modern algebraic notation. The rank of an operator is called its precedence, and an operation with a higher precedence is performed before operations with lower precedence. These rules are formalized with a ranking of the operators. In mathematics and computer programming, the order of operations is a collection of rules that reflect conventions about which operations to perform first in order to evaluate a given mathematical expression. Not to be confused with Operations order. Hello World § hello.zig const std = "std") pub fn main() ! void. To learn more about the Zig Standard Library, You will see many examples of Zig's Standard Library used in this documentation. Zig's Standard Library contains commonly used algorithms, data structures, and definitions to help you build programs or libraries. The Zig Standard Library has its own documentation. This HTML document depends on no external files, so you can use it offline.
#Explicit formula for arithmetic sequence guided notes code
The code samples in this document are compiled and tested as part of the main test suite of Zig. It isĪll on one page so you can search with your browser's search tool.
#Explicit formula for arithmetic sequence guided notes how to
This documentation shows how to use each of Zig's features. Often the most efficient way to learn something new is to see examples, so Resilient to changing requirements and environments. The language imposes a low overhead to reading code and is Maintainable Precisely communicate intent to the compiler and Reusable The same code works in many environments which have differentĬonstraints. Optimal Write programs the best way they can behave and perform. Robust Behavior is correct even for edge cases such as out of memory. Zig is a general-purpose programming language and toolchain for maintaining Cast Negative Number to Unsigned Integer.

Type Coercion: Compile-Time Known Numbers.Type Coercion: Slices, Arrays and Pointers.Type Coercion: Integer and Float Widening.String Literals and Unicode Code Point Literals.


 0 kommentar(er)
0 kommentar(er)
